Mathematics is important in everyday life. It is integral to all aspects of life and with this in mind we endeavour to ensure that all children develop a healthy and enthusiastic attitude towards mathematics that will stay with them.“
Welcome
Welcome to maths at Huntingdon Academy. On this page you will find out the key information about the leadership, design and implementation of our mathematics provision.
 | |
| Miss Forrest – Mathematics lead |
Maths at Huntingdon is lead by Miss Forrest.
As a maths leader, I believe that mathematics is essential to the children’s daily lives and a firm understanding in all areas of mathematics will benefit our children through their school years and into their future. Our maths curriculum aids children in making sense of the numbers, patterns and shapes they encounter in their environment, offers methods for handling data in an increasingly digital world and significantly contributes to their growth as successful learners. Our goal is to ensure every child at Huntingdon thrives in all areas of mathematics!
Our maths governor is Rachel Lewis.
Ours maths subject ambassadors are Ehsan (2N), Hamza (3C), Lilia (3W), Maleek (4K), Liren (5O), Rayan (5O), Laris (6A), Shayan (6L)
Subject Overview
At Huntingdon Academy, we believe that all pupils can succeed and achieve in mathematics! Indeed, we believe it to be the right of all students and it is important that all children regardless of their ability are given appropriate challenge and opportunity.
The intention of the maths curriculum at Huntingdon Academy is to equip children with confidence, resilience and a depth of understanding in maths that will underpin and indeed fuel their future learning. We aim to convey the belief to students that by working hard at mathematics they can succeed and that making mistakes is an essential and a valuable opportunity for new learning. It is also our aim, that through developing a strong home school partnership, we can help parents and carers to understand how they too can influence their children’s progress in maths.
Our approach at Huntingdon is under pinned by the principles of Mastery Maths, as we believe that this approach encourages a deep and connected understanding of mathematical ideas and concepts. We aim for our pupils to achieve Mastery through developing their procedural fluency and conceptual understanding.
We know that talking about maths helps embed and develop mathematical thinking and understanding. This aspect of teaching and learning is a particular focus at Huntingdon Academy due to the percentage of pupils with English as an additional language and those entering school with literacy levels below that of which is expected. In maths we endeavour to steep the maths curriculum that we deliver in language rich opportunities, to help the pupils’ ability to express their thinking about maths through talk.
National Curriculum Links
Conceptual Knowledge Maps
Year group planning
Calculation policy
Addition and Subtraction Policy
Multiplication and Division Policy
Pedagogy
At Huntingdon, our lessons follow a consistent structure designed to provide a framework or structure that teachers use to plan and design their daily lessons to ensure that elements of a good lesson are always included. This familiar structure allows pupils to build confidence and lessens time needed to explain the structure freeing up thinking for the maths.
Our lesson structure is as follows:
Flashback – Discover Task – Share and Think – Practice – Reflect
At Huntingdon, we plan and resource every maths lesson around the 5 Big Ideas.
The 5 Big Ideas:
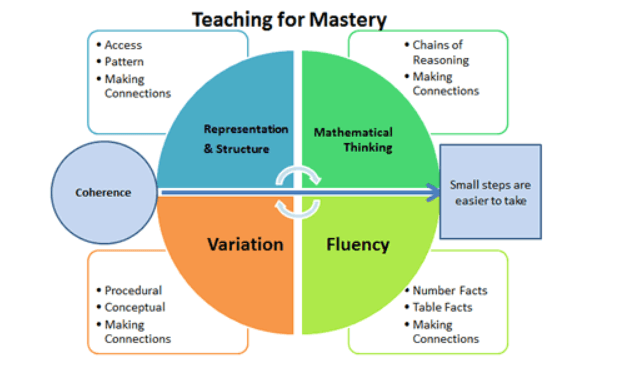
- Coherence
- A comprehensive, detailed conceptual journey through the mathematics.
- Connecting new ideas to concepts that have already been understood, and ensuring that, once understood and mastered, new ideas are used again in next steps of learning, all steps being small steps.
- Small steps – the teacher provides the steps but the child takes and connects the steps, reasoning along the way.
- Opportunities for depth.
- Stem sentences to support reasoning.
2. Representation and structure
- Mathematics is an abstract subject, representations have the potential to provide access and develop understanding.
- Representations and resources help to build concepts, draw attention to and expose the structure of the concept being taught.
- Moves from concrete, to pictorial, to abstract.
- One of the keys to mastery is exposing structure, however, to stop artificial early success – we often assume that our ‘rapid-graspers’ can already do, so don’t need exposure to such things. In fact it is only through such exposure that they are able to build a deep understanding.
3. Variation
- Conceptual variation draws attention to what it is, whereas non-conceptual variation draws attention to what it is not.
- Procedural variation draws attention to mathematical relationships and how they are connected through carefully chosen examples, which avoid mechanical repetition. Procedural variation is a careful choice of what to vary and careful choice of what the variation will draw attention to.
- This deepens their understanding further and allow them to create their own generalisations. This leads to intelligent practice.
- Research suggests that teaching through deliberate variation problems appears to be more effective than teaching through repeated explanations of a definition.
- Tasks should be designed in a way that avoids mechanical thinking so that children do not stop thinking. They should be taught to look for and recognise connections. Questions such as, What’s the same? What’s different? What do you notice?
4. Fluency
- Quick and efficient recall of facts and procedures and the flexibility to move between different contexts and representations of mathematics.
- Key facts such as number facts and times tables must be learnt and practiced regularly in order to avoid cognitive overload in the working memory. This helps students to focus on new ideas and concepts.
- The ability to recognise relationships/structures and make connections in mathematics.
5. Mathematical Thinking
- If taught ideas are to be understood deeply, they must not merely be passively received but must be worked on by the student: thought about, reasoned with and discussed with others.
- Pattern spotting allows pupils to make connections between mathematical relationships, notice the structure and to reason logically. Through this they also develop their ability to explain and convince others of their ideas.
- The quality and variety of language that pupils hear and speak are key factors in developing their mathematical vocabulary and presenting a mathematical justification, argument or proof. They must be assisted in making their thinking clear to themselves as well as others and teachers should ensure that pupils build secure foundations by using discussion to probe and remedy their misconceptions.
What teaching and learning looks like at Huntingdon Academy:
Talking:
Mathematical talk allows children to articulate, explain and develop their thinking through discussion and shared thinking.
We encourage students to ‘speak like a mathematician’ – which is to use mathematical vocabulary accurately and precisely, as well as to speak in full sentences when giving an explanation or answer.
E.g, a pupil would respond to the question, ‘What is 3 + 4 ?’ with ‘3 + 4 is 7’, rather than responding with just the answer ‘7’.
Vocabulary linked to the current concept is always displayed on the working wall.
Sentence stems and supports are visible on slides to encourage pupils to use full sentences.
Questioning:
We use questions that will help us to assess the students understanding. This is crucial in determining the next steps in planning and in identifying those that require further support and intervention. Knowing this will ensure that we act swiftly in order that all pupils keep up. Good questions also promote a deeper level of thinking which helps the students make mathematical links and connections as well as stimulate curiosity about the maths.
Modelling:
Teachers using the concrete, pictorial and abstract approach (CPA) to ensure that procedural and conceptual understanding are developed simultaneously. It is essential that this process is modelled to children so that they then have the skills to use these independently.
Reasoning:
Emphasis is placed on ‘learning’ through reasoning, developing multiple strategies and concepts towards understanding. Reasoning through a problem is powerful in helping to embed learning and enables the student to clearly share their thinking. As teachers this is invaluable in assessing a pupils true understanding.
Manipulatives:
In every classroom concrete resources, such as Base 10, Numicon and place value counters, will be used before moving learning into the pictorial and abstract. This allows children to become confident in their use and understanding of the number system. The will also be a range of visuals available such as number squares, multiplication grids and number lines. Different manipulatives are used to show the same concept. This exposes the students to the underlying mathematics so that they understand what is happening and do not become to associate a particular tool with a particular concept.
Mindset:
We encourage resilient, curious, collaborative, resourceful mathematicians and celebrate mistakes where they lead to learning.
Children are encouraged to;
- take pride in their work and present it in a way that is neat and can be clearly understood by others.
- work together and build on one another’s ideas, challenging their own and others thinking.
- take risks in their learning and be comfortable with taking challenges that extend thinking.
- Reflect on their learning and the learning
Challenge for all:
For those students who grasp ideas quickly, acceleration into new content is avoided. Instead, these students are challenged by deeper analysis of the lesson content and by applying the content in new and unfamiliar problem-solving situations.